Chapter 10 Review

• An Annuity is an account into which a sequence of scheduled payments is made.
• An Ordinary Annuity is an annuity into which equal payments are made at regular intervals, with the interest compounded at the end of each interval and with fixed interest rate for each compounding period.
  • $ A=\frac{p((1+\frac{r}{n})^{nt}-1)}{\frac{r}{n}}$
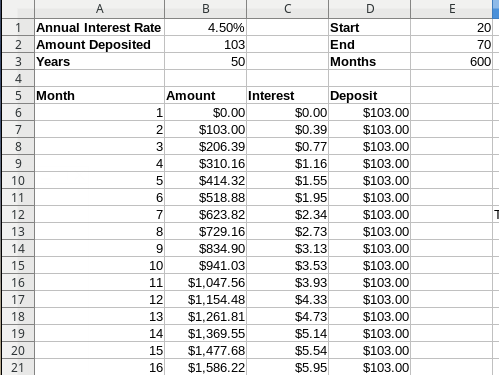
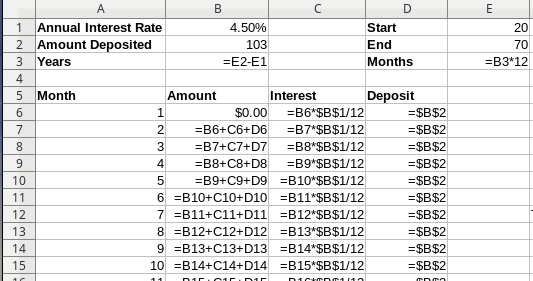
  • Build a spreadsheet
  • 
  • 
• Percent
  • Remember $\frac{1}{2}$% is not .5, it is .005. Why?
  • Percent: $\frac{part}{whole}\times 100\% = percent$
  • Percent change is
    • $\frac{new-old}{old}\times100\%$
• Simple Interest
  • $i=prt$
  • $A = i + p$
  • Normally 1 year = 360 days.
  • r and t must be in the same units.
  • Discount loans need to be worked twice
    • you are given p, r and t, find i
    • Calculate a new p = p-i
    • using i, p and t find r
• Compound interest
  • Interest earned on principal and interest
  • $A = p(1+\frac{r}{n})^{nt}$, or future value
  • $p=\frac{A}{(1+\frac{r}{n})^{nt}}$, or present value
• Installment loans
  • Use the appropriate table or
  • $m=\frac{p(\frac{r}{n})}{1-(1+\frac{r}{n})^{-nt}}$
  • $u=\frac{nPV}{100+V}$
  • Tables will be provided on the test.
  • For short term installment loans,
    • compute the monthly payment given r, n, p
    • compute r given the total amount.
    • Sometimes compute the unearned interest for paying off a loan early
    • final payment = $p + np -u$,
  • For mortgage
    • Compute down payment.
    • Compute monthly payment
    • Compute cost of points
    • Compute total amount of loan
    • Compute the first month interest.